Question

3 + x, 9 + 3x, 13 + 4x, ... is an arithmetic sequence for some real number x.

a. Find the value of x.

Answer 1

x = -2

Explanation:

An arithmetic sequence is one where the difference between any two consecutive terms in the sequence is constant.

For any arithmetic sequence the general formula is as follow

`a_(n) = a_(1) + (n - 1)d`

where

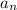`a_(n)` is the nth arithmetic value

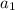`a_(1)` is the initial arithmetic value

`n` is the nth term

`d` is the difference is consecutive arithmetic values

To find the value of
`x` we form a number of simultaneous equations

`a_(1) = 3 + x\\ a_(2) = (3 + x) + 1 d\\ a_(3) = (3 + x) + 2 d`

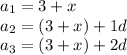
from the given information we also know that

`a_(2) = 9 + 3 x\\  a_(3) = 13 + 4x`

Solving these equations simultaneous we find the value of x to be

`x = -2`