Question

For a sample of eight​ bears, researchers measured the distances around the​ bears' chests and weighed the bears. Minitab was used to find that the value of the linear correlation coefficient is requals0.882. Using alphaequals​0.05, determine if there is a linear correlation between chest size and weight. What proportion of the variation in weight can be explained by the linear relationship between weight and chest​ size?

Answer 1

There is a linear correlation between the chest size and weight

Proportion of the variation in weight can be explained by the linear relationship between weight and chest​ size is 0.006724 .

Explanation:

Null Hypothesis:
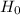`H_0`= There is no linear correlation between the chest size and weight

Alternative Hypothesis :
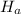`H_a`=There is a linear correlation between the chest size and weight

The value of the linear correlation coefficient is r=0.882.

n = 8

Formula :
`t=(r√(n-2))/(√(1-r^2))`

`t=(0.882√(8-2))/(√(1-(0.882)^2))`

`t=4.584`

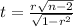
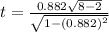
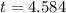
Degree of freedom = n-1 = 8-1 = 7

α =0.05

so, p value is 2.365

t calculated > t critical

So, we reject the null hypothesis

So, There is a linear correlation between the chest size and weight

The proportion of the variation in weight can be explained by the linear relationship between weight and chest​ size =
`r^2 = (0.082)^2=0.006724`

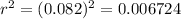
Hence proportion of the variation in weight can be explained by the linear relationship between weight and chest​ size is 0.006724 and There is a linear correlation between the chest size and weight