Question

Choose all the factors of 2x2−8x−642x2-8x-64

2x

(x-4)

(x+4)

(x+8)

(x+2)

x

2

(x-8)

Answer 1

2 is a factor

Explanation:

Here, the given equation is
`2x^(2)  - 8x -642x^(2)  -8x -64`

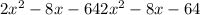
Check for 2x

Here, the last term has no x terms, so 2x is not a factor of the equation.

Check for (x-4)

Put x -4 =0, or x = 4 in equation

the answer ≠ 0, So, (x-4) is not a factor.

Check for (x+4)

Put x +4 =0, or x = -4 in equation

the answer ≠ 0, So, (x+4) is not a factor.

Check for (x+8)

Put x +8 =0, or x = -8 in equation

the answer ≠ 0, So, (x+8) is not a factor.

Check for (x+2)

Put x +2 =0, or x = -2 in equation

the answer ≠ 0, So, (x+ 2) is not a factor.

Check for (x)

Here, the last term has no x terms, so x is not a factor of the equation.

Check for 2

Here, the every term has even terms, so 2 is a factor of the equation.