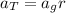Answer: The speed is not constant and the object is performing a non uniform circular motion
Step-by-step explanation:
Let's begin by explaining that the centripetal force is proportional to the centripetal acceleration
 of an object moving in circular motion is given by the following equation:
of an object moving in circular motion is given by the following equation:

Where:
 is the velocity
is the velocity
 is the radius of the circle
is the radius of the circle
In uniform circular motion, the centripetal acceleration vector is always perpendicular to the velocity vector, hence, the speed (the magnitude of velocity vector) is constant.
However, if a component of the centripetal acceleration vector is not perpendicular (is parallel to the velocity vector): the speed is not constant, the net force acting on the object will not be perpendicular to its motion and we will be dealing with non uniform circular motion.
It is important to note that in this situation the motion needs a tangential force, as well. Being the tangential acceleration
 proportional to
proportional to
 and the angular acceleration
and the angular acceleration
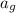 :
: