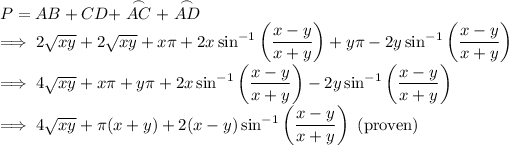Question

The diagram shows the cross-section of two cylindrical metal rods of radii x cm and y cm. A thin band, of length P cm, holds the two rods tightly together.
`\sf Show \: that\: P = 4√(xy) + \pi (x+y)+2(x-y)\:sin^(-1)\left((x-y)/(x+y) \right)`.

Answer 1

See below

Explanation:

Refer to the attachment (i)

Observe that

• 
  `P=AB+CD+\stackrel{\frown}{AC}+\stackrel{\frown}{BD}`
• 
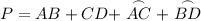
  `\Delta EFH` and
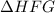  `\Delta HFG` are right angle triangles as well as congruent
• Rectangle ABFE is congruent to Rectangle GFDC

Consider ∆EFH

• HF(hypotenuse)=x+y
• EH(opposite side)=x-y

So,

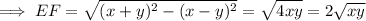`\displaystyle\implies EF=√((x+y)^2-(x-y)^2)=√(4xy)=2√(xy)` . . . . . using Pythagorean theorem.

`\displaystyle \sin(\theta)=(x-y)/(x+y)\\\implies \theta=\sin^(-1)\left((x-y)/(x+y)\right)`

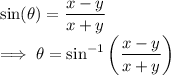
. . . . . using trigonometry

Now we can easily deduce that

• AB=2√xy
• CD=2√xy

Notice that

• 
  `\angle \stackrel{\frown}{AD}=\pi-2\theta`
• 
  `\angle \stackrel{\frown}{AC}=\pi+2\theta`

Recall that

`l_(arc)=\theta r`

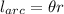
Therefore,

`\stackrel{\frown}{AC}=x\pi+2x\sin^(-1)\left((x-y)/(x+y)\right)\\\stackrel{\frown}{BD}=y\pi-2y\sin^(-1)\left((x-y)/(x+y)\right)`

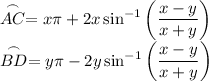
So,

`P=AB+CD+\stackrel{\frown}{AC}+\stackrel{\frown}{AD}\\ \implies 2√(xy)+2√(xy)+x\pi+2x\sin^(-1)\left((x-y)/(x+y)\right)+y\pi-2y\sin^(-1)\left((x-y)/(x+y)\right)\\ \implies 4√(xy)+x\pi+y\pi +2x\sin^(-1)\left((x-y)/(x+y)\right)-2y\sin^(-1)\left((x-y)/(x+y)\right)\\ \implies 4√(xy)+\pi(x+y)+2(x-y)\sin^(-1)\left((x-y)/(x+y)\right)\text{ (proven)}`