Answer:
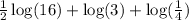 = log(3)
= log(3)
Explanation:
Given equation:
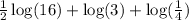
now,
we know the properties of log function as:
1) log(A) + log(B) = log(AB)
2) log(A) - log(B) =

3) log(Aᵇ) = b × log(A)
therefore,
using property 3 we get

or
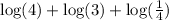
now,
using the property 2 we get
⇒ log(4) + log(3) + log(1) - log(4)
or
⇒ log(3) + log(1)
now,
using the property 1, we get
⇒ log(3 × 1)
or
⇒ log(3)