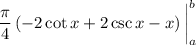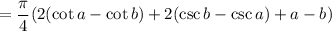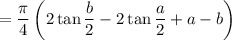It's unclear what the planes are supposed to be, so I'll take
 and
and
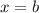 with
with
 .
.
The cross sections are disks with diameter
 , so each disk of thickness
, so each disk of thickness
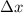 has a volume of
has a volume of

Then taking infinitesimally thin disks, we find the solid has a volume of
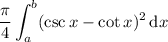
Since
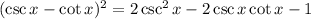
and
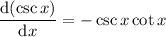
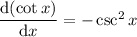
it follows that the volume is