Answer:
Let a and b integers and suppose that are even numbers. Then, exist integers k and m such that
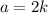 and
and
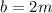 .
.
Then,
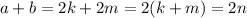
Since, k and m are integers then n=k+m is an integer. Then, since a + b is of the form 2 by an integer n, then a + b is an even number.