Question

Hudson travels 1080 miles in a jet and then 240 miles by car to get to a business meeting. The jet goes 300 mph faster then the rate of the car, and the car ride takes 1 hour longer then the jet. What is the speed of the car?

Answer 1

The speed of the car is 60 miles per hour.

SOLUTION:

Given, Hudson travels 1080 miles in a jet and then 240 miles by car to get to a business meeting.

Distance travelled in plane = 1080 miles

Distance travelled in car = 240 miles

The jet goes 300 mph faster than the rate of the car

Let the speed of plane be x and speed of car be y

x = 300 + y

y = x - 300 → (1)

And the car ride takes 1 hour longer then the jet.

Let the time taken by plane be m and time taken by car be n.

Then, n = 1 + m → (2)

We know that, distance traveled = speed
`*` time taken.

Now for plane, 1080 = x × m

xm = 1080 → (3)

Now for car, 240 =
`y * n`

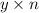
240 = (x – 300)(1 + m)

240 = x(1 + m) – 300( 1 + m)

240 = x + xm – 300 – 300m

x + xm – 300m – 300 – 240 = 0

x + 1080 – 300m – 540 = 0 [by using xm value in (3)

x – 300m + 540 = 0

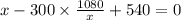`x-300 * (1080)/(x)+540=0` [ by using xm value in (3)]

`\begin{array}{l}{\mathrm{x}^(2)-300 * 1080+540 \mathrm{x}=0} \\ {\mathrm{x}^(2)+540 \mathrm{x}-300 * 3 * 360=0} \\ {\mathrm{x}^(2)-(360-900) \mathrm{x}-900 * 360=0}\end{array}`

Now, by comparing the above equation with general quadratic equation
`\mathrm{x}^(2)-(\mathrm{a}+\mathrm{b}) \mathrm{x}+\mathrm{ab}=0`

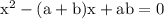
We can say that, a = 360 and b = -900

As speed cannot be negative, let us ignore b

So, x = 360.

Substitute x value in (1)

y = 360 – 300 = 60

hence, the speed of the car is 60 miles per hour.