Answer:
The probability is 0.046
Solution:
As per the question:
Minimum calorie intake of an individual per day,
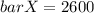
The no. of calories ingestion on an average per day per capita, Mean
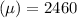
Standard deviation,
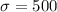
No. of individuals in a random sample, n = 36
Now,
The Probability for the sample mean to exceed the WHO minimum is given by:
P(
![\bar{X} geq 2600) = [tex]\frac{\bar{X} - \mu}{(\sigma)/(n)}](https://img.qammunity.org/2020/formulas/health/high-school/wqeuyqr22d1xuhtk9j9r4ckw6tuhpuy1c5.png)
P(
/((500)/(6)) = 1.68](https://img.qammunity.org/2020/formulas/health/high-school/xud6jxp7ulasa1y31ixttc005ey36vzu91.png)
Now, with the help of Standard Normal table:
P(Z > 1.68) = 0.0046