Question

A waitress sold 1818 ribeye steak dinners and 2424 grilled salmon​ dinners, totaling ​$579.85579.85 on a particular day. Another day she sold 3030 ribeye steak dinners and 88 grilled salmon​ dinners, totaling ​$581.35581.35. How much did each type of dinner​ cost?

Answer 1

The cost of each ribeye steak dinners is $14.20 and the cost of each grilled salmon​ dinners is $19.43

Step-by-step explanation:

The correct question is

A waitress sold 18 ribeye steak dinners and 24 grilled salmon​ dinners, totaling ​$579.85 on a particular day. Another day she sold 30 ribeye steak dinners and 8 grilled salmon​ dinners, totaling ​$581.35 How much did each type of dinner​ cost?

Let

x ----> the cost of each ribeye steak dinners

y ----> the cost of each grilled salmon​ dinners

we know that

`18x+24y=579.85` ----> equation A

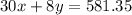`30x+8y=581.35` -----> equation B

Solve the system by graphing

Remember that the solution of the system of equations is the intersection point both graphs

The solution is the point (14.20,19.43)

see the attached figure

therefore

The cost of each ribeye steak dinners is $14.20 and the cost of each grilled salmon​ dinners is $19.43