Answer:
a)Dimensions of pool A are length = 6.667m and width = 3.667 m and dimension of pool B are length = 7.333m and width = 3.333m.
b) Area of pool A is equal to area of pool B equal to 24.44 meters.
Solution:
Let’s first calculate area of pool A .
Given that width of the pool A = (x+3)
Length of the pool A is 3 meter longer than its width.
So length of pool A = (x+3) + 3 =(x + 6)
Area of rectangle = length x width
So area of pool A =(x+6) (x+3) ------(1)
Let’s calculate area of pool B
Given that length of pool B is double of width of pool A.
So length of pool B = 2(x+3) =(2x + 6) m
Width of pool B is 4 meter shorter than its length,
So width of pool B = (2x +6 ) – 4 = 2x + 2
Area of rectangle = length x width
So area of pool B =(2x+6)(2x+2) ------(2)
Since area of pool A is equal to area of pool B, so from equation (1) and (2)
(x+6) (x+3) =(2x+6) (2x+2)
On solving above equation for x
(x+6) (x+3) =2(x+3) (2x+2)
x+6 = 4x + 4
x-4x = 4 – 6
x =

Dimension of pool A
Length = x+6 = (
 ) +6 = 6.667m
) +6 = 6.667m
Width = x +3 = (
 ) +3 = 3.667m
) +3 = 3.667m
Dimension of pool B
Length = 2x +6 = 2(
 ) + 6 =
) + 6 =
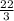 = 7.333m
= 7.333m
Width = 2x + 2 = 2(
 ) + 2 =
) + 2 =
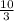 = 3.333m
= 3.333m
Verifying the area:
Area of pool A = (
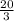 ) x (
) x (
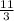 ) =
) =
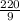 = 24.44 meter
= 24.44 meter
Area of pool B = (
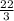 ) x (
) x (
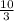 ) =
) =
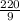 = 24.44 meter
= 24.44 meter
Summarizing the results:
(a)Dimensions of pool A are length = 6.667m and width = 3.667 m and dimension of pool B are length = 7.333m and width = 3.333m.
(b)Area of pool A is equal to Area of pool B equal to 24.44 meters.