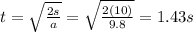Question

platform diving in the olympic games takes place at two heights: 5 meters and 10 meters. What is the velocity of a diver entering the water from each platform if he steps off the platform initially? How much time does it take the diver to reach the water from each platform?

Answer 1

1) Velocity: 9.9 m/s and 14 m/s

The motion of the diver is a free-fall motion, so it is a uniform accelerated motion. Choosing downward as positive direction, the final velocity can be found by using the following suvat equation:

`v^2-u^2=2as`

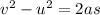
where

v is the final velocity

u = 0 is the initial velocity (the diver starts from rest)

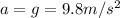`a=g=9.8 m/s^2` is the acceleration of gravity

s is the displacement

For the diver jumping from 5 m, s = 5 m, so

`v=√(2as)=√(2(9.8)(5))=9.9 m/s`

For the diver jumping from 10 m, s = 10 m, so

`v=√(2as)=√(2(9.8)(10))=14 m/s`

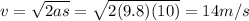
2) Time: 1.01 s and 1.43 s

The time of flight of each diver can be found by using the other suvat equation

`s=ut+(1)/(2)at^2`

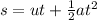
And since u = 0, it can be reduced to

`s=(1)/(2)at^2`

For the diver jumping from 5 m, s = 5 m, so we find

`t=\sqrt{(2s)/(a)}=\sqrt{(2(5))/(9.8)}=1.01 s`

For the diver jumping from 10 m, s = 10 m, so we find

`t=\sqrt{(2s)/(a)}=\sqrt{(2(10))/(9.8)}=1.43 s`