Question

Determine the wavelength of the photon associated with the transition in which the electron in a hydrogen atom goes from n = 6 to n = 4 a photon with a wavelength of 2694 nm is released a photon with a wavelength of 2694 nm is absorbed a photon with a wavelength of 2625 nm is absorbed a photon with a wavelength of 2533 nm is released a photon with a wavelength of 2625 nm is released

Answer 1

a photon with a wavelength of 2625 nm is released

Step-by-step explanation:

`E_n=-2.179* 10^(-18)* (1)/(n^2)\ Joules`

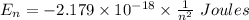
For transitions:

`Energy\ Difference,\ \Delta E= E_f-E_i =-2.179* 10^(-18)((1)/(n_f^2)-(1)/(n_i^2))\ J=2.179* 10^(-18)((1)/(n_i^2) - (1)/(n_f^2))\ J`

`\Delta E=2.179* 10^(-18)((1)/(n_i^2) - (1)/(n_f^2))\ J`

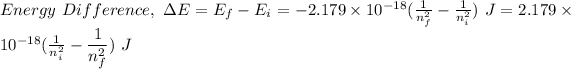
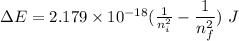
Also,
`\Delta E=\frac {h* c}{\lambda}`

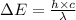
Where,

h is Plank's constant having value
`6.626* 10^(-34)\ Js`

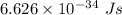
c is the speed of light having value
`3* 10^8\ m/s`

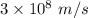
So,

`\frac {h* c}{\lambda}=2.179* 10^(-18)(|(1)/(n_i^2) - (1)/(n_f^2)|)\ J`

`\lambda=\frac {6.626* 10^(-34)* 3* 10^8}{{2.179* 10^(-18)}* (|(1)/(n_i^2) - (1)/(n_f^2)|)}\ m`

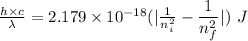
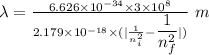
So,

`\lambda=\frac {6.626* 10^(-34)* 3* 10^8}{{2.179* 10^(-18)}* (|(1)/(n_i^2) - (1)/(n_f^2)|)}\ m`

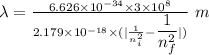
Given,
`n_i=6\ and\ n_f=4`

`\lambda=\frac{6.626* 10^(-34)* 3* 10^8}{{2.179* 10^(-18)}* ((1)/(6^2) - (1)/(4^2))}\ m`

`\lambda=(10^(-26)* \:19.878)/(10^(-18)* \:2.179\left(|(1)/(36)-(1)/(16)\right)|)\ m`

`\lambda=(19.878)/(10^8* \:2.179\left(|-(5)/(144)\right|))\ m`

`\lambda=2.625* 10^(-6)\ m`

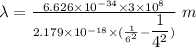
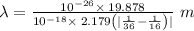
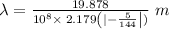
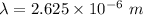
1 m = 10⁻⁹ nm

`\lambda=2625\ nm`

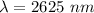
From coming from higher energy level to lower, energy is released.

Hence, correct option is - a photon with a wavelength of 2625 nm is released