Question

The flow of a river at the start of a baseflow recession, on June 7, 2018, was 4,790 t3/s. After 59 days of continuous recession, on August s, 2018, the flow had declined to 1,631 t/s. (a) What is the value of the recession constant? (b) What will the flow be on September 12, 2018, if the recession continues?

Answer 1

recession constant = 0.9819

Q(97) = 815.6910 m³/s

Step-by-step explanation:

given data

on June 7, 2018

flow = 4790 m³/s

august flow declined = 1,631 m/s

time = 59 day from June 7, 2018 i.e on August 5, 2018

time = 97 days from June 7, 2018 i.e on September 12, 2018

solution

we know here equation that is

Q(t) = Q(o)
`e^(-at)`

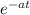
here Q(t) is flow at any time t and Q(o) is initial flow and

K is recession constant i.e
`e^(-a)`

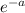
so first we find recession constant

Q(t) = Q(o)
`e^(-at)`

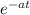
put here value

1631 = 4790
`e^(-a(59))`

take ln both side

-1.07733 = - 59 a

a = 0.01825

so

recession constant =
`e^(-a)`

recession constant =
`e^(-0.01825)`

recession constant =
`e^(-0.01825)`

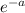
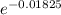
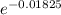
recession constant = 0.9819

and

flow after 97 day that is on 12 September

Q(97) = 4790
`e^(-0.01825(97))`

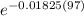
Q(97) = 815.6910 m³/s