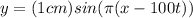Question

Two waves are generated on a string of length 3.0 m to produce a three-loop standing wave with an amplitude of 1.0 cm. The wave speed is 100 m/s. Let the equation for one of the waves be of the form y(x, t) # ym sin(kx " vt). In the equation for the other wave, what are (a) ym, (b) k, (c) v, and (d) the sign in front of v?

Answer 1

For the other wave, ym = 0.30 cm, k = 4.30 m^-1, v = 3 s^-1, and the sign in front of v is negative.

Step-by-step explanation:

In the equation for the other wave:

(a) ym = 0.30 cm, as the amplitude of both waves is the same.

(b) k = 4.30 m^-1, as the wave number remains the same for both waves.

(c) v = 3 s^-1, as the wave speed is the same for both waves.

(d) The sign in front of v is negative, as one wave is moving in the opposite direction from the other wave.

Answer 2

Part a)

`y_m = 1 cm`

Part b)

`k = \pi rad/m`

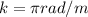
Part c)

v = 100 m/s

Part d)

since wave is moving in +x direction

so here the sign must be negative

so complete wave equation is

`y = (1 cm) sin(\pi(x - 100t))`

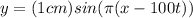
Step-by-step explanation:

As we know that the wave equation is given as

`y = y_m sin(k(x`

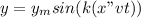
here we know that

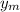`y_m` = maximum displacement of the particle

Part a)

maximum displacement = amplitude

so here we know that

`y_m = 1 cm`

Part b)

k =
`(2\pi)/(\lambda)`

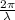
here we know that length of the string is 3 m

it consist of 3 loops

so we will have

`3 (\lambda)/(2) = 3 m`

`\lambda = 2 m`

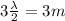
so we have

`k = (2\pi)/(2)`

`k = \pi rad/m`

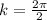
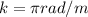
Part c)

v = wave speed

v = 100 m/s

Part d)

since wave is moving in +x direction

so here the sign must be negative

so complete wave equation is

`y = (1 cm) sin(\pi(x - 100t))`