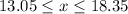Answer with explanation:
Given : A poll found that a particular group of people read an average of 15.7 books per year.
i.e.
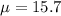
The pollsters are 99% confident that the result from this poll is off by fewer than 2.65 books from the actual average x.
i.e. E= 2.65
The confidence interval for population mean is given by :-
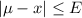
Hence, the required inequality involving absolute value for :
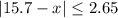
To solve this inequality for x, we have

Hence, the interval in which the average is likely to fall :