Answer: a)
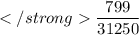 b)
b)
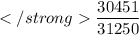
Explanation:
Since we have given that
Number of days in a year = 365
Number of students = 50
Probability that no two students share the same birthday. It means each student has different birthday.
Probability(no two students share the same birthday)
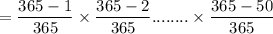
Probability(no two students share the same birthday)
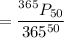
Probability(no two students share the same birthday)

Probability that at least one of the students has the same birthday as another student in the class is given by
