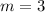Question

The graph of 4x+12y=8 and y=Mx+5 are perpendicular. What is the value of m

Answer 1

`m=3`

Explanation:

we know that

If two lines are perpendicular, then their slopes are opposite reciprocal (the product of their slopes is equal to -1)

step 1

Find the slope of the given line

we have

`4x+12y=8`

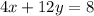
isolate the variable y

`12y=8-4x`

`y=(2)/(3)-(1)/(3)x`

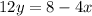
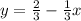
the slope of the given line is

`m=-(1)/(3)`

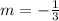
step 2

Find the slope
`m_2` of the perpendicular line to the given line

`m_1*m_2=-1`

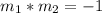
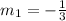`m_1=-(1)/(3)` ---> slope of the given line

`(-(1)/(3))*m_2=-1`

`m_2=3`

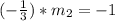
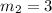
therefore

The value of m is

`m=3`