Answer:
126 miles per hour
Explanation:
We can draw a triangle and we can use trigonometry to write:
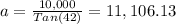
Where a is the distance plane flew in 1 minute
Since, 11,106.13 FEET in 1 MINUTE, to get in hours, we multiply by 60:
11,106.13 * 60 = 66,367.51 feet per hour
Now, to get in miles per hour, we divide by 5280 [5280 feet = 1 mi]
66,367.51 / 5280 = 126.21 miles per hour
To the nearest mph, it would be 126 miles per hour