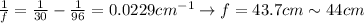Question

What is the focal length of a concave mirror that magnifies, by a factor of +3.2, an object that is placed 30 cm from the mirror?

A. 23cm
B. 32cm
C. 44cm
D. 46cm

Answer 1

C. 44 cm

Step-by-step explanation:

We can solve the problem by using the lens equation:

`(1)/(f)=(1)/(p)+(1)/(q)`

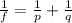
where

f is the focal length

p = 30 cm is the distance of the object from the mirror

q is the distance of the image from the mirror

We also know that the magnification is

`M=-(q)/(p)=+3.2`

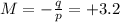
So

`q=-3.2p=-3.2(30)=-96 cm`

Substituting into the first equation, we find f:

`(1)/(f)=(1)/(30)-(1)/(96)=0.0229 cm^(-1) \rightarrow f=43.7 cm \sim 44 cm`