Answer:
 m
m
Step-by-step explanation:
Consider the motion of the boat across the stretch from west to east direction :
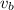 = velocity of boat = 15 mph
= velocity of boat = 15 mph
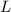 = length of the stretch = 1.01 miles
= length of the stretch = 1.01 miles
 = Time taken to reach the opposite end
= Time taken to reach the opposite end
Time taken to reach the opposite end is given as
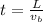
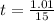
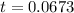 h
h
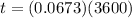 sec (Since 1 h = 3600 sec)
sec (Since 1 h = 3600 sec)
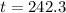 sec
sec
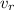 = speed of river = 2.04 m/s
= speed of river = 2.04 m/s
 = distance traveled downstream
= distance traveled downstream
Distance traveled downstream is given as


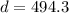 m
m