Answer:
The x one bedroom is 93 unit, y two-bedroom is 62 units, and z three-bedroom is 31 units.
Explanation:
Consider the provided information.
A total of 186 units are planned,
Apartment complex consisting of x one-bedroom units, y two-bedroom townhouses, and z three-bedroom townhouses.
This can be written as:
 ......(1)
......(1)
The number of family units (two- and three-bedroom townhouses) will equal the number of one-bedroom units.
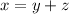 ......(2)
......(2)
The number of one-bedroom units will be 3 times the number of three-bedroom units,
 ......(3)
......(3)
From 3 and 2.

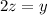
Substitute the value of x and y in equation 1.
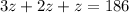

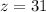
Substitute the value of z in equation 3
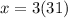

Substitute the value of z in
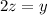
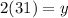

Thus, the x one bedroom is 93 unit, y two-bedroom is 62 units, and z three-bedroom is 31 units.