Question

PLEASE HELP ASAP!!

Part A: Explain why the x-coordinates of the points where the graphs of the equations y = 4−x and y = 2−x+1 intersect are the solutions of the equation 4−x = 2−x+1.

Part B: Make tables to find the solution to 4−x = 2−x+1. Take the integer values of x between −2 and 2.

Part C: How can you solve the equation 4−x = 2−x+1 graphically?

Answer 1

Solutions:

Part A:

Let
`y_(1)=(4-x) \text { and } y_(2)=(2-x+1)`

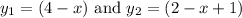
As we know that the values at the point of intersection are the values that satisfy both the equation at that particular point.

So at insertion point,
`y_(1)=y_(2)`

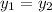
Hence
`(4-x)=(2-x+1)`

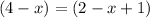
Part 2:

The problem asked to make tables to find the solution to
`4-x=2-x+1`

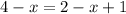
If we take the first equation,

`y=4-x`

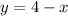
then, the table for (x,y) is
`(-2,6), (-1,5), (0,4), (1,3), (2,2), (3,1), (4,0)`

if we take the second equation,

`y=2-x+1`

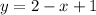
then the table for (x,y) is
`(-2,5),(-1,4), (0,3), (1,2), (2,1), (3,0), (4,-1)`

Part 3:

We can solve the equation
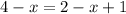`4-x = 2-x+1` graphically by drawing the line
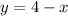`y = 4-x` and
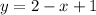`y= 2-x+1` in the graph. The intersecting point of both the lines will be the solution.