Question

Which scale would produce the smallest drawing

1: 100

1 Millimeter: 1meter

1 inch: 10 feet

Can SOMEBODY HELP ME

Answer 1

1 Millimeter: 1 meter

Explanation:

a) the scale is
`(1)/(100)`

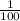
That means ----> 1 unit in the drawing represent 100 units in the actual

b) the scale is
`(1)/(1)(mm)/(m)`

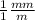
Remember that

1 m=1,000 mm

substitute

`(1)/(1,000)(mm)/(mm)=](1)/(1,000)`

That means ----> 1 unit in the drawing represent 1,000 units in the actual

c) the scale is
`(1)/(10)(in)/(ft)`

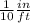
Remember that

1 ft=12 in

substitute

`(1)/(10)(in)/(ft)=](1)/(120)`

That means ----> 1 unit in the drawing represent 120 units in the actual

Example

If we would like to draw a segment that in reality measures 1000 units, then

with scale a)
`(1)/(100)`

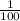
The length of the drawing is 1,000/100=10 units

with scale b)
`(1)/(1,000)`

The length of the drawing is 1,000/1,000=1 unit

with scale c)
`(1)/(120)`

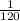
The length of the drawing is 1,000/120=8.3 units

therefore

The smallest drawing is with the scale of
`(1)/(1,000)`

so

1 Millimeter: 1 meter