Question

An opera singer in a convertible sings a note at 750 Hz while cruising down the highway at 89 km/h . The speed of sound in the air is 343 m/s. What is the frequency heard by a person standing beside the road in front of the car? What is the frequency heard by a person standing beside the road behind the car? Express your answer with the appropriate units.

Answer 1

In front of the car: f=808.3Hz

Behind the car: f=699.6Hz

Step-by-step explanation:

The Doppler formula is:

`f=(v \pm v_r)/(v \pm v_s)f_0`

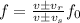
Where f is the observed frequency,
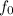`f_0` the emitted one, c the speed of sound on that medium,
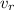`v_r` the velocity of the receiver relative to the medium (positive if the receiver is moving towards the source, negative otherwise) and
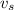`v_s` the velocity of the source relative to the medium (positive if the source is moving away from the receiver, negative otherwise).

First we convert the speed of the car (which is the source) to S.I.:

`v_s=89km/h=89(km)/(h)(1000m)/(1km)(1h)/(3600s)=24.72m/s`

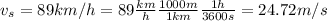
Then we can substitute our values (noticing that the receiver is not moving relative to the medium):

`f=(v)/(v \pm v_s)f_0=((343m/s))/((343m/s) \pm (24.72m/s))(750Hz)`

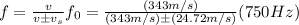
So for the frequency heard by a person standing in front of the car we need to use the negative sign since the source is moving towards the receiver, and for the frequency heard by a person standing behind the car we need to use the positive sign since the source is moving away from the receiver.

Then,

For the person in front of the car: f=808.3Hz

For the person in behind the car: f=699.6Hz