Answer:
Step-by-step explanation:
We can solve this problem using the ideal gas law
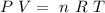
where P is the pressure, V the volume, n the number of moles, R the ideal gas constant and T the temperature.
We can use the atmospheric pressure as 1 atm, and the body temperature as 36.5 °C, in Kelvin this is:
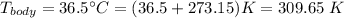
The ideal gas constant is:
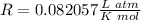
taking all this in consideration, the number of moles will be:
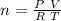
![n = \frac{1 \ atm * 3.8 \ L }{ 0.082057 (L \ atm)/( K \ mol)]() * 309.65 \ K } [/tex]
* 309.65 \ K } [/tex]
