Question

A 0.5-cm diameter air bubble at 5°C and 3 atm pressure rises to the surface. Determine its final diame- ter when T 25°C and P 1 atm. (Ignore any water vapor in the bubble.)

Answer 1

d=0.738cm

Step-by-step explanation:

We divide this problem in two parts, below the surface is part 1 (where we have
`P_1V_1=n_1RT_1`), and on the surface part 2 (where we have
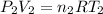`P_2V_2=n_2RT_2`). In this case, pressure, volume and temperature will vary, and n will remain constant because the amount of air is always the same (and R is always a constant), so we have
`n_1=n_2`.

We can then do:

`nR=(P_1V_1)/(T_1)=(P_2V_2)/(T_2)`

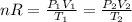
The volume of a sphere is
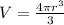`V=(4\pi r^3)/(3)`, so we can write

`(P_1)/(T_1)(4\pi r_1^3)/(3)=(P_2)/(T_2)(4\pi r_2^3)/(3)`

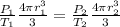
Which is

`(P_1r_1^3)/(T_1)=(P_2r_2^3)/(T_2)`

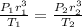
And obtain our radius for the bubble on the surface:

`r_2=\sqrt[3]{(P_1T_2r_1^3)/(P_2T_1)}=\sqrt[3]{(P_1T_2)/(P_2T_1)}r_1`

Since the diameter is 2 times the radius this can be written as (the factor 2 cancels out):

`d_2=\sqrt[3]{(P_1T_2)/(P_2T_1)}d_1`

And we put our values in S.I.:

`d_2=\sqrt[3]{((3atm)(298K))/((1atm)(278K))}(0.005m)=0.00738m=0.738cm`