Step-by-step explanation:
Let the diameter of the Sun in the model be = x
Scale used in the model =
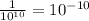
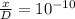
The real diameters of the Sun ,D= 1.4 million kilometers =
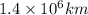
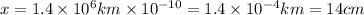
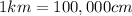
The diameter of the sun in the model is 14 cm.
The real diameters of the Earth,d = 12,800 km
Let the diameter of the Earth in the model be = y
Scale used in the model =
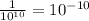
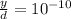
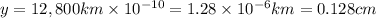
The diameter of the Earth in the model is 0.128 cm.
The Earth-Sun distance ,l =
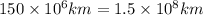
Let the Earth-Sun distance in the model be = z
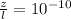

The distances between Earth-Sun in the model is 1500 cm.