Answer:
The anwers are:
a) The slope field is attached.
b) The general solution is
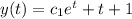
c) The solution that it is exactly a straight line is y(t)=t+1 (when c1=0)
Explanation:
y'(t)=y-t
y'(t)-y=-t
First we find the solution of the homogenous equaiton:
y'(t)-y=0
Considering
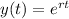 where r is a constant
where r is a constant
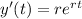
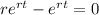
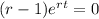
 is never zero, so:
is never zero, so:
(r-1)=0
r=1

The particular solution is given by:
y(t)=At+B
y'(t)=A
Hence,
y'(t)-y=-t
A-At-B=-t
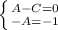
A=C=1
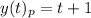
The general solution is the sum of y(t)h and y(t)p:
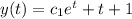
When c1=0, y(t)=t+1 which is a straight line of slope 1 and intercept 1.