Answer:
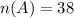
Explanation:
In sets theory n(A) represents the number of elements in set A.This number is the cardinal number of the set A.
For n(AUB) there is an equation that relates n(A),n(B) and n(A∩B) :
n(AUB) = n(A) + n(B) - n(A∩B)
In our exercise :
n(AUB) = n(A) + n(B) - n(A∩B)
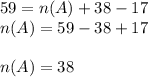
Another important equation for maths is similar to this one :
P(AUB) = P(A) + P(B) - P(A∩B)
Where ''P'' is the event probability.This equation is used in probability and statistics.