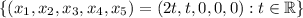Answer:
The system to solve is
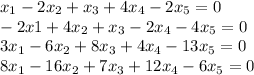
and the augmented matrix of the system is
![\left[\begin{array}{cccccc}1&-2&1&4&-2&0\\-2&4&1&-2&-4&0\\3&-6&8&4&-13&0\\8&-16&7&12&-6&0\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/on7jtgpp2obrmnderbme63byfehotl238b.png)
Using row operations we obtain the echelon form of the matrix, that is,
![\left[\begin{array}{ccccccc}1&-2&1&4&-2&0\\0&0&-1&-20&10&0\\0&0&0&-54&22&0\\0&0&0&0&13&0\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/ag16tcb6tjz2enxpk4wwh64qu6hywp84pa.png)
Now, we use backward substitution:
1.
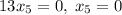
2.

3.
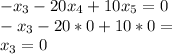
4.

The system has infinite solutions and the set of solutions is: