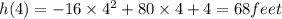Question

The height h, in feet, of a ball that is released 4 feet above the ground with an initial velocity of 80 feet per second is a function of the time t, in seconds, the ball is in the air and is given by

h(t)=-16t^2+80t+4, 0 < t < 5.04

a. Find the height of the ball above the ground 2 seconds after it is released.

b. Find the height of the ball above the ground 4 seconds after it is released.

Please show work!

Answer 1

Part a)

Height of ball after 2 seconds it is released is

h(2)=-16\times 2^2+80\times 2+4=100feet

Part b)

Height of ball after 4 seconds it is released is

h(4)=-16\times 4^2+80\times 4+4=68feet

Explanation:

Answer 2

Answer with Step-by-step explanation:

The height of the ball from the ground as a function of time is given by

`h(t)=-16t^2+80t+4`

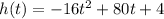
The height of the ball at any instant of time can be found by putting the value of time 't' in the above relation as

Part a)

Height of ball after 2 seconds it is released is

`h(2)=-16* 2^2+80* 2+4=100feet`

Part b)

Height of ball after 4 seconds it is released is

`h(4)=-16* 4^2+80* 4+4=68feet`