Question

Given the formula for an arithmetic sequence f(9) = f(8) + 5 written using a recursive formula, write the sequence using an arithmetic formula.

f(9) = f(1) + 5
f(9) = f(1) + 30
f(9) = f(1) + 35
f(9) = f(1) + 40

Answer 1

f(9)=f(1)+40

Explanation:

We are given term=previous term+5 by the equation f(9)=f(8)+5 and that the sequence is arithmetic.

This tells the slope of the line is 5. (We know it is linear because it is arithmetic).

So we want to find the slope of the line going through (1,f(1)) and (9,f(9)).

`(f(9)-f(1))/(9-1)=5`

`f(9)-f(1)=5(9-1)`

`f(9)-f(1)=5(8)`

`f(9)-f(1)=40`

Answer 2

Option D.

Explanation:

The recursive formula for an arithmetic sequence

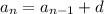`a_n=a_(n-1)+d` .... (1)

where, nis the number of term and d is the common difference.

Given the recursive formula for an arithmetic sequence

`f(9)=f(8)+5` .... (2)

From (1) and (2) we get

`n=9,d=5`

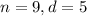
The explicit formula for an arithmetic sequence is

`a_n=a_1+(n-1)d`

where, a1 is first term and d is common difference.

Substitute n=9 and d=5 in the above formula.

`a_9=a_1+(9-1)(5)`

`a_9=a_1+40`

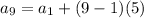
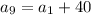
It can be written as

`f(9)=f(1)+40`

Therefore, the correct option is D.