Answer:
a)H=84.87 m
b)R=565.27 m
Step-by-step explanation:
Given that
Speed of ball on earth
U=41.2 m/s
θ=31°
We know that
Maximum height in projectile motion given as
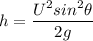
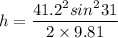
h=22.94 m
Range in projectile motion given as
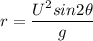
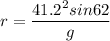
r=152.77 m
Given that on distance planet ball moves 3.7 times far more as on earth.
So on the distance planet
The maximum height ,H=3.7 h
H= 3.7 x 22.94
H=84.87 m
The range on distance planet
R=3.7 r
R=3.7 x 152.77 m
R=565.27 m