Question

With one method of a procedure called acceptance​ sampling, a sample of items is randomly selected without replacement and the entire batch is accepted if every item in the sample is okay. A company has just manufactured 1969​CDs, and 400are defective. If 3of these CDs are randomly selected for​ testing, what is the probability that the entire batch will be​ accepted? Does this outcome suggest that the entire batch consists of good​ CDs? Why or why​ not?If 3of these CDs are randomly selected for​ testing, what is the probability that the entire batch will be​ accepted?The probability that the whole batch is accepted is nothing.​(Round to three decimal places as​ needed.)Does the result in​ (a) suggest that the entire batch consists of good​ CDs? Why or why​ not?A.​No, because only a probability of 1 would indicate the entire batch consists of good CDs.B.​Yes, because it is not unlikely that the batch will be accepted.C.​No, because the sample will always consist of good CDs.

Answer 1

a) 95%

b) No, because the sample size is too small.

Explanation:

There are

`\binom{1969}{3}=(1969!)/(3!(1969-3)!)=1,270,351,544`

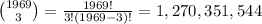
ways of choosing 3 elements out of 1969 without repetition.

By the rule of product, there are

400*399*388 = 63,520,800

ways of selecting 3 defective CD's

So, the probability of taking 3 defective CD's out of 1969 is

63,520,800/1,270,351,544 = 0.05 (5%)

Hence, the probability of choosing 3 non defective CD's is

0.95 (95%)

And this is the likelihood the entire batch will be accepted.

This result should not indicate that the entire batch consists of good CD's because the sample size is too small.

Determining the sample size depends on several factors which include the margin of error acceptable and the size of the population.

But as a rule of thumb, for a population less than 10,000 a 10% is considered a good size of sample.

So, in this case, a sample should consist of around 190 CD's.