Question

Which equation models the rational function shown in the

graph?
f(x) = 2(x+2)/x-2
f(x) = x-2/x+2
flv) = 2(x-2)
f(x) = x+2/x-2

Answer 1

2(x+2)/x-2

Explanation:

Answer 2

f(x)=
`(2(x+2))/(x-2)`

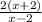
Explanation:

We are given that a graph.

We have to find the equation which models the rational function shown in the graph.

From given graph we can see that

At x=2, the function in the graph is approaches to infinity.

It means function is not defined at x=2

We know that a rational function is undefined when denominator is equal to zero.

f(x)=
`(2(x+2))/(x-2)`

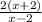
x-2=0

x=2

It means function is not defined at x=2

y-intercept:y=-2

The function is passing through the point (1,-6).

When substitute x=1

`f(1)=(2(1+2))/(1-2)=-6`

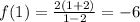
Therefore,Option 2 is not correct because function is not defined at x=-2

Hence, option 1 is true.