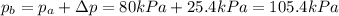Question

Oil with density of 0.8505 and velocity of 4m/s in a pipe that has a downward slope of 1:50 (sine). At a certain point in the pipe, a pressure gauge shows a pressure of 80kPa.

Determine the pressure at a point 200m downstream of the gauge if:
(a) The flow losses are ignored (ideal case);
(b) There is a flow loss equal to 10% of the total initial head.

Answer 1

(a) Pressure at point 200 m downstream: 113.4 kPa

(b) Pressure at point 200 m downstream: 105.4 kPa

Step-by-step explanation:

The point of reference, that will be named Point A, is the pressure gage.

The point 200 m downstream will be named Point B.

With a slope of 1:50, the height difference between A and B is (1/50)*200=4m.

(a) In the case there is no friction losses, we can write

`p_a+(\rho V^2)/(2) +\rho*g*h_a=p_b+(\rho V^2)/(2) +\rho*g*h_b`

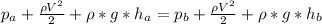
Because ther is no change in the diameter of the pipe, V=constant.

Rearranging

`p_a+\rho*g*h_a=p_b+\rho*g*h_b\\\\\Delta p = \rho * g * \Delta h\\\\\Delta p = 0.8505 * 1000(kg)/(m^3) *9.81(m)/(s^2)*4 m\\\\ \Delta p = 33.373.62 kg/(m*s^2)=33.373.62 Pa=33.4 kPa`

Then we have an increase in of 33.4 kPa, and pressure in Point B (downstream) is:

`p_b=p_a+\Delta p = 80 kPa + 33.4 kPa = 113.4 kPa`

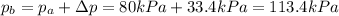
(b) If we consider 10% of the total initial head as friction loss, we have

`p_a+\rho*g*h_a=p_b+hf +\rho*g*h_b\\\\hf=0.1*p_a\\\\\Delta P = \rho*g*\Delta h-0.1*p_a\\\\\Delta P = 0.8505*1000 kg/m^3*9.81m/s2*4m-0.1*80kPa\\\\\Delta P = 33.4kPa-8kPa=25.4kPa`

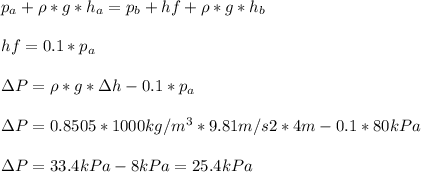
In this case the rise in pressure is 25.4 kPa, due to the friction losses.

The pressure at point B is

`p_b=p_a+\Delta p = 80 kPa + 25.4 kPa = 105.4 kPa`