Answer:
Force will become
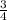 times.
times.
Step-by-step explanation:
We have given two charge of opposite sign that are held at a fixed distance
According to coulombs law force between two charges is given by

let initially the charges are
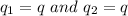
So force
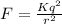
Now according to question half of the charge is transferred to second charge particle
So
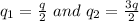
As the distance remain unchanged
So force

So
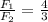

So force will become
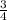 times.
times.