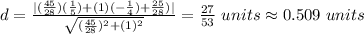Question

Find the distance between point ( 1/5 , − 1/4 ) and line y=− 45/28 x− 25/28

Answer 1

`distance=(27)/(53)\ units\approx0.509\ units`

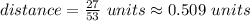
Explanation:

You need to use the following formula for calculate the distance between a point and a line:

`d=( |Ax_1+By_1+C|)/(√(A^2+B^2))`

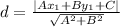
Rewrite the equation of the given line in the following form:

`Ax+By+C=0`

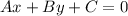
Then:

`(45)/(28)x+y+(25)/(28)=0`

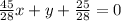
You can identify that:

`A=(45)/(28)\\\\B=1\\\\C=(25)/(28)`

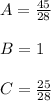
Given the point:

`((1)/(5),-(1)/(4))`

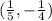
You can identify that:

`x_1=(1)/(5)\\\\y_1=-(1)/(4)`

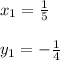
Therefore, you can substitute values into the formula:

`d=\frac {\sqrt{((45)/(28))^2+(1)^2}}=(27)/(53)\ units\approx0.509\ units`