Answer: The voltage of the cell is 0.80 V.
Step-by-step explanation:
The given cell is:
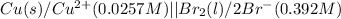
Half reactions for the given cell follows:
Oxidation half reaction:
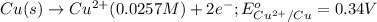
Reduction half reaction:
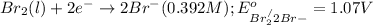
Net reaction:

Oxidation reaction occurs at anode and reduction reaction occurs at cathode.
To calculate the
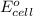 of the reaction, we use the equation:
of the reaction, we use the equation:
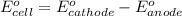
Putting values in above equation, we get:
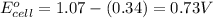
To calculate the EMF of the cell, we use the Nernst equation, which is:
![E_(cell)=E^o_(cell)-(0.059)/(n)\log ([Cu^(2+)]* [Br^(-)]^2)](https://img.qammunity.org/2020/formulas/chemistry/college/oev4xr8l6d9sopcnvkwol3qymy23gt2a93.png)
where,
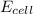 = electrode potential of the cell = ?V
= electrode potential of the cell = ?V
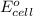 = standard electrode potential of the cell = +0.73 V
= standard electrode potential of the cell = +0.73 V
n = number of electrons exchanged = 2
![[Cu^(2+)]=0.0257M](https://img.qammunity.org/2020/formulas/chemistry/college/8otwjhi8qatzpg11md3siuasl4n7r1orjc.png)
![[Br^(-)]=0.392M](https://img.qammunity.org/2020/formulas/chemistry/college/azgotkumy5eokju0e2zagnh8b3f4dsill3.png)
Putting values in above equation, we get:
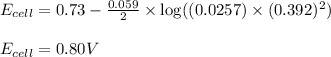
Hence, the voltage of the cell is 0.80 V.