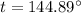Question

An aluminum alloy rod has a length of 6.3243 cm at 16.00°C and a length of 6.3568 cm at the boiling point of water. (a) What is the length of the rod at the freezing point of water? (b) What is the temperature if the length of the rod is 6.3689 cm?

Answer 1

a)
`6.318\ \rm m`

b
`144.89^\circ`

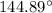
Step-by-step explanation:

Given :

• Length of the aluminium alloy at
  `16^\circ`
  `=L_1=6.3243\ \rm \ m`
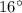
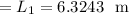
• Length of the aluminium alloy at
  `100^\circ`
  `=L_2=6.3568\ \rm \ m`

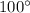
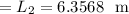
Let
`\alpha` be the coefficient of linear thermal expansion of aluminium alloy

When the temperate of the rod is increased then its length will be changed accordingly. Let
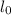`l_0` be its length at freezing point of water at T=
`0^\circ`

`l_1=l(1+\alpha \Delta T)\\\\(l_1)/(l)-1=\alpha \Delta T`

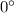
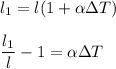
According to question we have

a)

`(6.3243)/(l)-1=\alpha (16-0)\\(6.3568)/(l)-1=\alpha (100-0)`

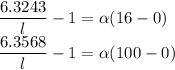
Solving above two equations we get

`l=6.318\ \rm m`

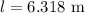
b) Let
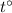`t^\circ` be the temperature of the rod when its length is 6.3689 m then we have

`(6.3689)/(6.2838)-1=\alpha(t-0)`

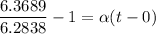
Also we have

`(6.3243)/(6.318)-1=\alpha (16-0)`

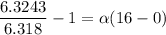
Solving above two equations we have

`t=144.89^\circ`