Question

A test of the prototype of a new automobile shows that the minimum distance for a controlled stop from 95 km/h to zero is 55 m. Find the acceleration, assuming it to be constant as a fraction of the free-fall acceleration. The acceleration due to gravity is 9.81 m/s 2 . Answer in units of g

Answer 1

-0.64525g

Step-by-step explanation:

t = Time taken for the car to stop

u = Initial velocity = 95 km/h

v = Final velocity = 0 km/h

s = Displacement

a = Acceleration

Equation of motion

`v^2-u^2=2as\\\Rightarrow a=(v^2-u^2)/(2s)\\\Rightarrow a=(0^2-95^2)/(2* 0.055)\\\Rightarrow a=-82045.45\ km/h^2`

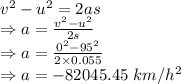
Converting to m/s²

`a=82045.45=(82045.45* 1000)/(3600* 3600)=-6.33\ m/s^2`

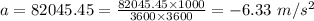
g = Acceleration due to gravity = 9.81 m/s²

Dividing both the accelerations, we get

`(a)/(g)=(-6.33)/(9.81)=-0.64525\\\Rightarrow a=-0.64525g`

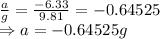
Hence, acceleration of the car is -0.64525g