Question

When measuring the length a and the width b of a rectangle, it was found that 5.4< a< 5.5 and 3.6< b< 3.7 (in centimeters). Find the possible values of: the area of the rectangle

Answer 1

The possible values of: the area of the rectangle are all real numbers greater than 19.44 square centimeters and less than 20.35 square centimeters

`19.44\ cm^2< A< 20.35\ cm^2`

Explanation:

Let

a ----> the length of rectangle

b ----> the width of rectangle

we know that

The area of rectangle is

`A=ab`

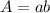
we know that

`5.4\ cm< a< 5.5\ cm\\3.6\ cm< b< 3.7\ cm`

Determine the maximum area and the minimum area

Maximum area

For a=5.5 cm, b=3.7 cm

`A<(5.5)(3.7)`

`A<20.35\ cm^2`

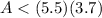
Minimum area

For a=5.4 cm, b=3.6 cm

`A>(5.4)(3.6)`

`A>19.44\ cm^2`

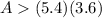
so

`19.44\ cm^2< A< 20.35\ cm^2`

therefore

The possible values of: the area of the rectangle are all real numbers greater than 19.44 square centimeters and less than 20.35 square centimeters