Answer:

Step-by-step explanation:
Given:
- Electric field just outside the given sphere, E = 459 N/C.
- Inner radius of the shell,
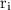 = 10 cm = 0.1 m.
= 10 cm = 0.1 m. - Outer radius of the shell,
 = 20 cm = 0.2 m.
= 20 cm = 0.2 m. - Electric field outside the shell after introducing the charge Q,
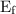 = 181 N/C.
= 181 N/C.
Before introducing the charge Q,
Consider a Gaussian sphere of same radius as the outer radius of the shell, concentric with the given shell,
Applying Gauss' law over this surface,
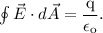
where,
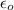 is the electrical permittivity of free space having value
is the electrical permittivity of free space having value
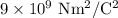 .
.
 is the surface area element of the sphere, directed along the normal to the plane of the surface.
is the surface area element of the sphere, directed along the normal to the plane of the surface.
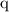 is the net charge enclosed by the Gaussian surface.
is the net charge enclosed by the Gaussian surface.
The directions of the area element and the electric field, both are directed outward the surface, therefore,
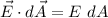 .
.
The LHS of the equation of the Gauss' law is given as
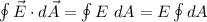
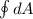 is the surface area of the Gaussian sphere =
is the surface area of the Gaussian sphere =
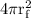
Therefore, using the equation of the Gauss' law,
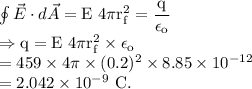
It is the net charge enclosed by the outer surface before Q was introduced.