Answer:
(a) 677.49 N
(b)
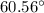
Step-by-step explanation:
Given:
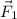 = force in the first rope = 333 N west =
= force in the first rope = 333 N west =
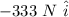
 = force in the second rope = 590 N south =
= force in the second rope = 590 N south =
Assume:
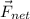 = force in a single rope
= force in a single rope
 = angle with the west direction
= angle with the west direction
We know force as a vector quantity. The two forces acting on the heavy box will have a resultant force whose magnitude and direction will be equivalent to the force required in a single rope that would produce the same effect on the box.
Let us first try to find out the resultant force.
Since the resultant of a force is calculated by the vector addition of all the force vectors.
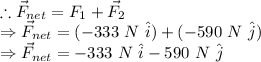
Part (a):
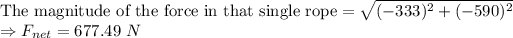
Hence, a force of 677.49 N should be applied by a single rope to do the same effect.
Part (b):
Since the resultant force vector is has its coordinates in the third quadrant of the cartesian vector plane. So, the vector will absolutely make a positive angle with the west direction which is given by:
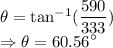
Hence, the rope should be at angle
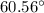 south of west.
south of west.