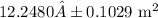Answer:
A)
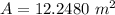
B)
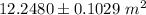
Step-by-step explanation:
Given:
Length of the room
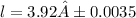
Width of the room
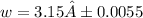
A) Let A be the area of the room
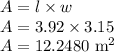
B)We will calculate uncertainty in each dimension
%uncertainty in length
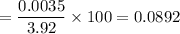
%uncertainty in width =
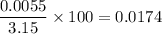
The uncertainty in area will be sum of uncertainty in length and width
%uncertainty in Area= %uncertainty in length + %uncertainty in width
%uncertainty in Area
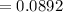
%uncertainty in Area=0.0106
Uncertainty in Area

There Area is