Question

Jim and Abby each bought burgers and fries from the concession stand at the fair. Jim bought 3 burgers and 2 orders of fries for $17. Abby bought 2 burgers and 4 orders of fries for $18. How much for each burger cost? How much for each order of fries?

Answer 1

Jim bought 3 burgers for $5.50 & 2 orders of fries for $3.00. Abby bought 2 burgers for $9.00 and 4 orders of fries for $5.00.

Explanation:

Answer 2

Answer: Each burger costs $4 and each order of fries costs $2.5 .

Explanation:

Let x be the cost of each burger and y be the cost of each orders of fries.

Given : Jim bought 3 burgers and 2 orders of fries for $17.

Abby bought 2 burgers and 4 orders of fries for $18.

Then, the system of linear equation will become :

`3x+2y=17-------(1)\\\\2x+4y=18-------(2)`

Divide by 2 on each sides of equation (2), we get

`x+2y=9-------(3)`

Eliminate equation (3) from (1), we get

`2x=8`

Divide by 2 on both sides , we get

x=4

Put value of x in (3), we get

`4+2y=9`

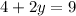
Subtract 3 both sides, we gte

`2y=5`

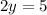
Divide by 2 both sides , we get

y=2.5

Hence, each burger costs $4 and each order of fries costs $2.5 .