Answer:
The maximum height is 162.67 m.
Step-by-step explanation:
Suppose the total height is h.
And at the height of 2/3h the speed of an object is,
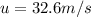
And the remaining height will be,
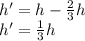
So, according to question the initial speed is,
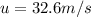
Acceleration in the upward direction is negative,
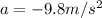
And the final speed will be v m/s which is 0 m/s.
Now according to third equation of motion.
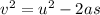
Here, v is the final velocity, u is the initial velocity, a is the acceleration, s is the displacement.

Therefore, the maximum height is 162.67 m.