Question

A policeman is chasing a criminal across a rooftop at 10 m/s. He decides to jump to the next building which is 2 meters across from the original and 2.5 meters lower. How far (in m) will they fall in that time? (use g = 10 m/s^2)

Answer 1

They will meet at a distance of 7.57 m

Given:

Initial velocity of policeman in the x- direction,
`u_(x) = 10 m/s`

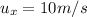
The distance between the buildings,
`d_(x) = 2.0 m`

The building is lower by a height, h = 2.5 m

Solution:

Now,

When the policeman jumps from a height of 2.5 m, then his initial velocity, u was 0.

Thus

From the second eqn of motion, we can write:

`h = ut + (1)/(2)gt^(2)`

`h = (1)/(2)gt^(2)`

`2.5 = (1)/(2)* 10* t^(2)`

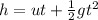
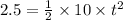
t = 0.707 s

Now,

When the policeman was chasing across:

`d_(x) = u_(x)t + (1)/(2)gt^(2)`

`d_(x) = 10* 0.707 + (1)/(2)* 10* 0.5 = 9.57 m`

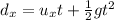
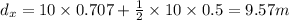
The distance they will meet at:

9.57 - 2.0 = 7.57 m